Mastering the crosswind
Author: Colin Aro
Article extracted from LightspeedAviation.com
The original article has been divided into four to facilitate the reader’s reading.
The Effect of a Crosswind
In chapter one, we talked about the keys to a good approach to landing – being stabilized at the appropriate speed, attitude, and configuration. Moving forward, we’ll add discussion of crosswinds and how to appropriately compensate using crabs and slips. Let’s begin by looking at the effect of a crosswind on an aircraft’s flight path. Consider an aircraft heading due north with a 10 knot wind 30 degrees to the left of heading (Figure 1).
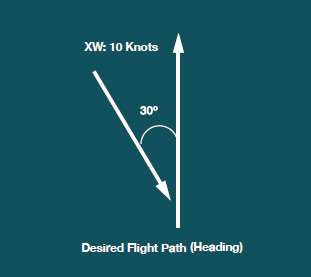
Of course, we analyze vectors by using simple trigonometry to break them into components, in this case headwind and crosswind components. Figure 2 shows the resulting component vectors: This 10 knot crosswind 30 degrees off of the aircraft’s nose has the effect of a 5 knot direct crosswind (crosswind component) and an 8.6 knot direct headwind (headwind component).
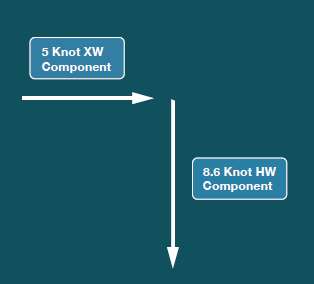
If we were landing in this wind condition, we can immediately see that crosswinds are not all bad: this one slows our groundspeed by almost 9 knots leading to a slower touchdown speed. However, we will also be drifting to the right at a speed of 5 knots. Bear in mind that 1 knot is about 100 feet per minute and 5 knots (500 feet per minute) is about 8 feet per second. Left uncorrected, this rate of sideways drift will have you off into the weeds after only 10 seconds or so even on a very wide runway. This is certainly quite a noticeable drift rate for a crosswind component with the strength of a gentle breeze. Ultimately, we are not pointed in the same direction we are flying and this will cause problems on touchdown even if we do stay out of the weeds.
OK, standby for some simple trigonometry: the crosswind component is calculated by multiplying the wind speed (10 knots in this case) by the sine of the crosswind angle (30 degrees in this case). I promise, that’s all you need to know. The sine of 30 degrees is 0.5 and so the crosswind component is
0.5 * 10 knots = 5 knots
There are a few rules of thumb that will help you estimate crosswind components to within a knot or two. As noted, the crosswind component for a 30 degree crosswind is half (exactly) the total wind velocity. This increases to about 70 percent of total velocity (approximately) at 45 degrees of crosswind and 87 percent at 60 degrees. So, for crosswinds 30 degrees or less, you can assume the crosswind component to be 25 – 50% of the total wind speed (the closer to 30 degrees, the closer to 50%). More than 35 degrees and you can conservatively expect the component to be 70 – 100% of the total wind speed (the farther past 45 degrees, the closer to 100%).
Landings aside, Figure 2 shows us that left uncorrected, the crosswind will drift the aircraft to the right of the desired course at a rate of 5 knots. The obvious way to correct for this is to “crab” or angle the aircraft’s flight path into the wind. The FAA even gives us a formula (“angle to parallel”) to quantify how much the aircraft’s heading needs to be angled in order to maintain flight path:
α = 60(δ)/D
where δ – crosswind component
D – airspeed
α – crab angle
This leads to a striking coincidence if the aircraft’s speed is 60 knots (not too far off the approach speed of many light GA aircraft): the equation reduces to
α = δ
That is, at 60 knots, the aircraft’s crab angle in degrees is roughly equal to the crosswind component in knots. At 120 knots, the crab angle is half the crosswind component and so forth. Not only does this give the pilot another way to estimate crosswind component (in the absence of a weather reporting station, no less), it shows us that even with a modest 5 knot crosswind component we’ll have approximately a 5 degree crab angle for a typical light GA aircraft. That’s a 5 degree difference between the direction the aircraft is pointed and the direction it is travelling. Crab angles are effective in maintaining ground track, but in terms of transitioning from the air to the ground, we still have some more work to do. Being pointed in a direction different than the direction of travel is a sure fire recipe for directional control problems upon touchdown. Especially if you’re flying a dreaded taildragger!
In the next chapter, we will discuss the forces on an aircraft in turning flight and apply this knowledge to crosswind slips to land.








